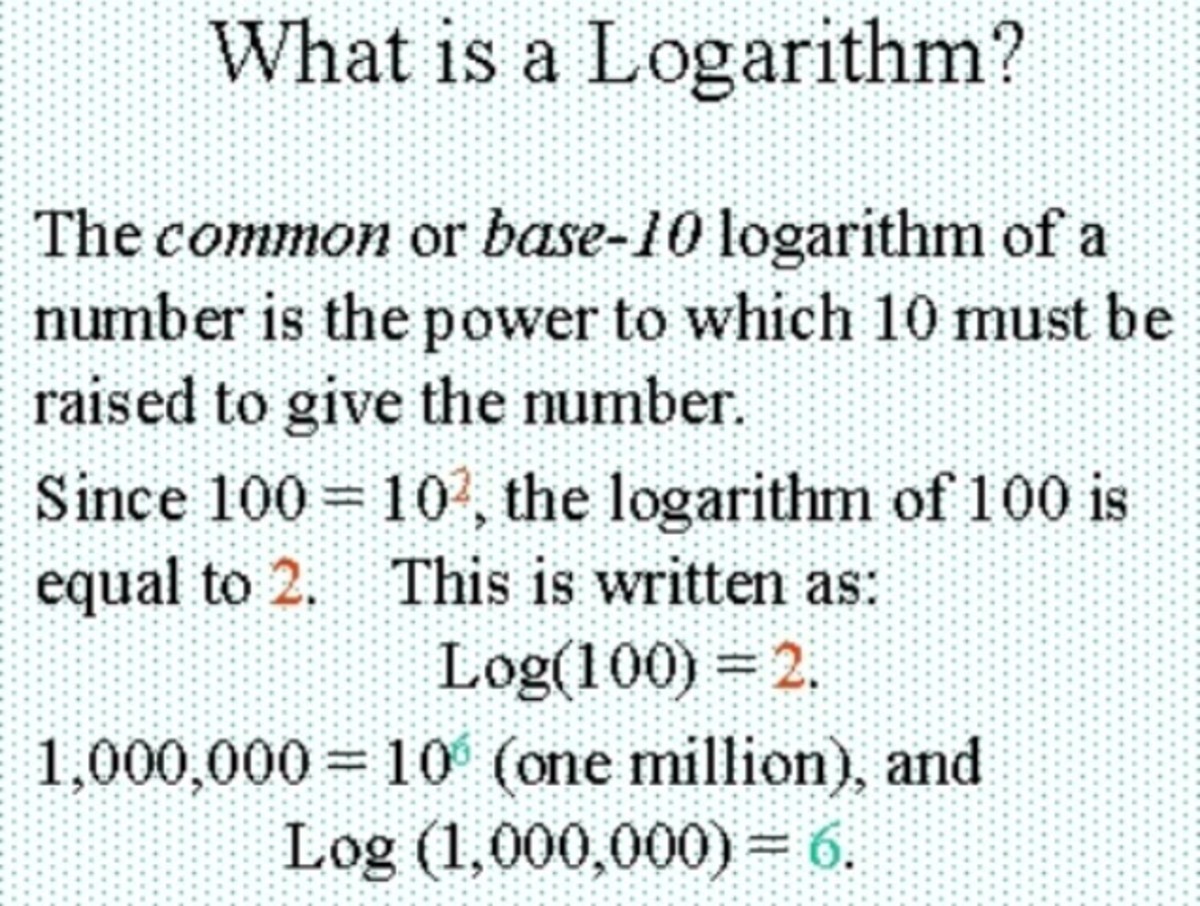
The dB (deciBel) and the Bel are formally recognized measurement parameters, with the lowly status of ‘Accepted non-S.I. units’, within the International System of Units. This system is the body responsible for establishing worldwide S.I. units, the units by which all things are ultimately measured. The ‘Accepted’ status is due to their historically wide usage and usefulness, otherwise they would probably be deprecated by the S.I. System. The Bel was originally developed for use in the telephone industry and was named in honor of the telephone inventor, Alexander Graham Bell. It was initially found to be very convenient for comparing two power levels, for reasons discussed later, but then proved to be too large for practical use and was replaced by the dB, which has been in general use since about 1925, and which, at 1/10th of a Bel, is more convenient and useful.
Why and When Is a dB Used?
Both terms (dB and Bel) were units of transmission measurement and were originally used to measure a signal level change, gain or loss, when the signal was ‘transmitted’ from its source to a receiver where it can be measured. The transmission can be of any format, either through the air, through a transmission line or cable, through an amplifier or other circuit, or through an item (attenuator) to lower the signal. Image by StereoSound from Pixabay A relatively easy way to describe a signal level change is to compare the output or received value with the input or source value and then to produce a ratio (A:B or A/B) of output to input. The input B, equivalent to a math denominator and, in ratios, mathematically termed the consequent, should ideally be referenced to 1 and then it is easy to state, for example, ‘a gain of 2x (2 times)’ or ‘a loss/reduction of half (0.5)’. However, with the advent of high gain amplifiers (op amps) with gains of 100-plus, and especially when they were connected in cascade or in series, the linear gain ratios became very large and unwieldy with figures of 100,000 and greater. The Bel and the dB are logarithmic terms and both convert these high gain vales to convenient, manageable, much lower figures and allow logarithmic amplifier gains to be easily added instead of multiplied. The smaller dB is the more convenient and useful of the two terms.
How Do We Make Use of a dB?
One way of utilizing the dB is probably of interest to many people, not only to electronics technicians and engineers, which is the listing of loudspeaker specifications, including the popular Bluetooth ‘speakers.
Exploring the Math
In the photo below: #2 states Signal-to-Noise Ratio: 84dB or higher#3 states Sensitivity: 80dB+2dB (Actually both are incorrectly ‘db’ instead of the official dB). Solving for PR (in the math equation (i) below) using the 84 S-to-N dB reveals a ratio of approximately 251x106 times, which actually means that the signal is some 251 million times greater than the noise level. This shows the convenience of the dB for quoting S/N ratios: 84 dB versus 251x106 times. Solving for the 80 dB Sensitivity, again in (i) below, shows a still very high but much lower ratio of 100 x106 times. This also shows another aspect of the dB in that a very large change in a power ratio (251 million versus 100 million) actually results in only a small change in the dB value. In fact a change of only +3dB means a doubling of the power and of only -3dB means a halving of the power.
In What Other Ways Is the dB Used?
When connecting amplifiers in series, or cascading them, or when removing them from the chain, it is easier to manipulate the dB values, by simple adding and subtracting, rather than having to multiply the linear gains. Sound engineers in bands and concerts use the loudspeaker dB specifications as necessary when connecting the equipment to attach the required cascaded amplifiers to the loudspeakers according to their sensitivities. Many areas of everyday life now also use some form of dB to quote measurements and specifications. However, the dB is termed a Relative or Relational measurement because it is a ratio of one level to another and is not a quantifiable parameter or what is termed an absolute reading or measurement e.g. Volts, Amps, etc.
The dBm and Other Absolute Levels
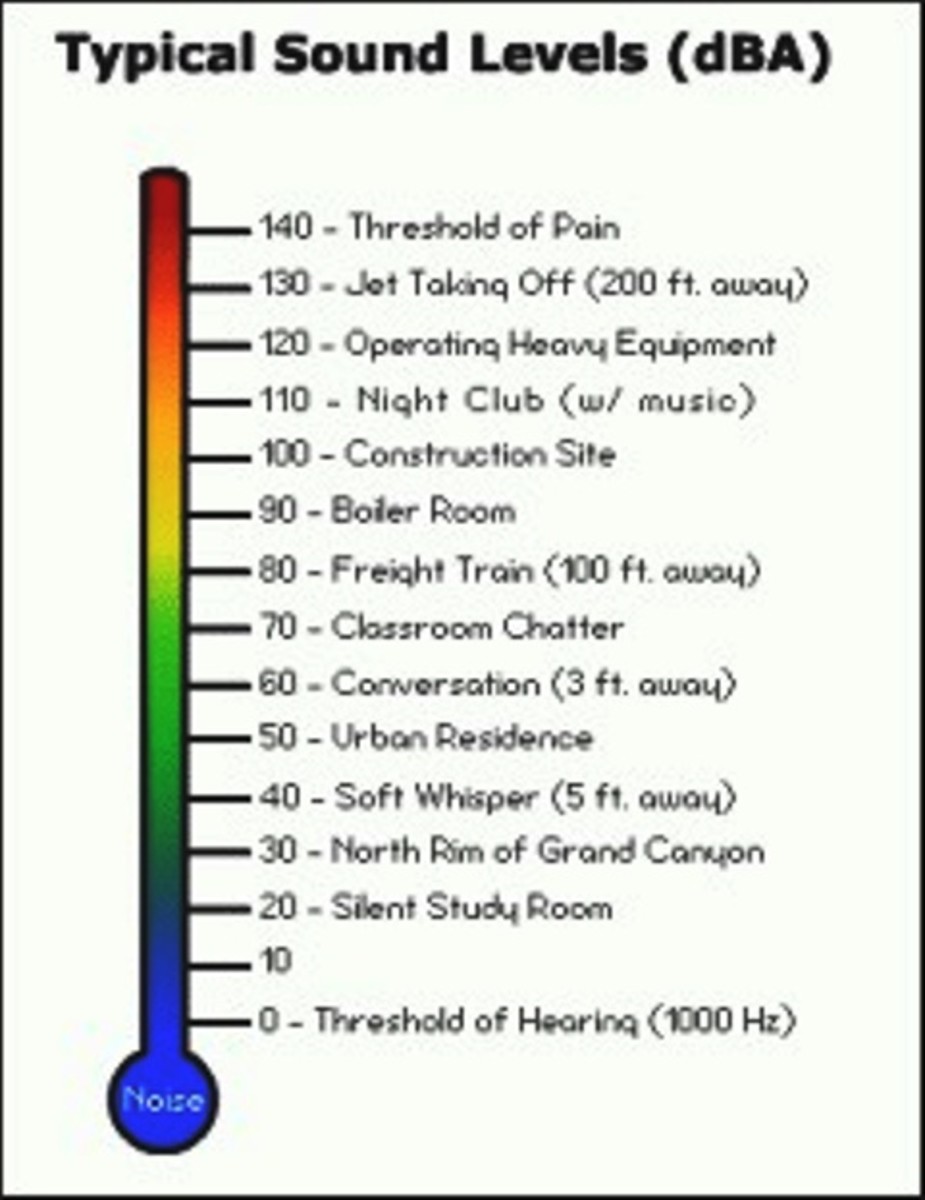
The absolute term used in power level measurements is the dBm. The measurements are ‘relative to’ or ‘with respect to’ (wrt) a 1mW reference level and so they are no longer simply related to input and output levels. The 1mW level is also the 0 dBm level as can be calculated with equation (i) below. There are other 0 dB reference levels in use other than 1 mW. High power engineers can use the dBk which is a 0 dB, 1 kV reference level. The dBV is a 0 dB reference level relative to 1 Volt. The dBA will be known to audio enthusiasts or to acoustic techs. The A could mean Audio or Acoustic but specifically refers to the A-weighted shaped audio frequency range filter that is used when testing sound and noise levels. The dBA is relative to the standard lower threshold of human hearing at 1 kHz. The dB (SPL) is another acoustic scale of measurement and reporting.
What Other Fields Use Logarithmic Progressions (Log.Progs.)?
The human ear responds logarithmically to sounds. There is a lot of amplification at low sounds levels and much less at high levels. So that, in simple terms, the barely heard level up to the damage level of the ear’s sound range is from the approximate 0 dB threshold (actually very slightly higher than zero) to about 130/140 dB which in reality is a range of greater than 2 million times. Hence volume controls often vary logarithmically to mimic the response of the human ear for a smoother sound control. These are more expensive than the cheaper linear or pseudo-log volume controls. Earthquake strengths are also logarithmic and not linear. The human eye responds logarithmically to brightness. There are several other Log.Prog. systems that a www search will find, particularly in Wikipedia.
Doing the Math
It is easy to work in dBs as the following table shows.
A doubling of any power level is a 3dB increase; a halving of power is a (-)3dB reduction. An increase of 10x (10-times) of any power level is an increase of 10dB and similarly a reduction of 10x is a (-)10dB reduction.
Only the 3dB and 10dB level changes can be obtained from the table or from the known relationship between dBs and power level changes. Other values can only be estimated by interpolating the table values or must be calculated using the given formulas below.
The Long Math
The math for when we are measuring output and input transmission power is: (i) dB = 10 x LOG10 Signal Power Ratio (PR) For when we are measuring output and input signal level voltage, the math is: dB = 20 x LOG10 Signal Voltage Ratio (VR) This content is accurate and true to the best of the author’s knowledge and is not meant to substitute for formal and individualized advice from a qualified professional. © 2019 Stive Smyth
Comments
Stive Smyth (author) from Philippines on December 07, 2019: Hi John. I’m glad that you enjoyed the parts that you could. I’m at a crossroads — I maybe need to “get the tech out of my system” before I can write more limericks, poems and articles of “other” interest. But, after forty-five years of being in tech, maybe I can only forever do both? Time will tell. Also high time that I did some more reading and not constant writing :) John Hansen from Australia (Gondwana Land) on December 07, 2019: Some of this is beyond my comprehension but I found the basics and background of dB and the Bel quite interesting. I appreciate the amount of work that went into this article, Stive. Thank you for sharing.